Bach was a blues person maybe
Was Bach a blues person? #
I wondered today, as I played the first few bars of Capriccio sopra la lontanza del fratello dilettissimo. Then I remembered that I played a little bit of the song on the guitar by Federico Carulli called Waltz, and to me it seemed similar to rock or soul music, I could even hear Janis Joplin sing in my mind.


🎲 dice reading #
inspired by Ellen Langer's idea on decisions, I now use dice to decide what to read, and it's fun and brings variability to learning.
for example if I have 6 books to read, then I can throw two dice and if it's 2, 3, then I read the third chapter of the second book. Or I can go 2 + 3, or 23, or 3 mod 2, 2•3, etc.
another idea of learning from textbooks #
that works well is to do the first exercise from the first book, then the first from the second, then then the second from the first, and so on. Computer agent suggested this diagonal approach: E_12 E_21 E_12 E_31 E_22 E_13 … E_91 E_82 E_73 … E_19
if you have 9 books, for example.
These two approaches seem to take off the stress of being stuck at pages. I often had the experience of staring at pages trying to reconnect with what I read, while my mind have wondered elsewhere.
"hard" mathematics concepts #
can be reframed as sea treasures 🐚🐙🪢, like loot in a video game because I had the experience of seeing these hard to understand pages as awful, "another page I don't understand", Yesterday for example when I started reading Calculus by Apostol I felt as if it was a story, a real book, "wow I'm really enjoying this, this time I might finally read an entire book about calculus and will understand it", like finally I was understanding what I was reading, until page 5, when I had no clue what I was reading.
Then I realised that this was a familiar experience, a pattern, that I experience with other mathematics books. "Oh I will read this book on topology! I love it, until page 3, when it's Proof:…"
But I also realised that if I persist and maybe put the page into Anki, and look up the same concept in other books, then I am often surprised that I can learn this stuff.
Also, after struggling a bit with the material, I can postpone it and read move on to other chapters, read the first paragraphs, look at figures, it is likely that the authors mention "remarkable", "fascinating", "miraculous", "beautiful", important connections to other things, geometric representations. Recently I have been struggling to understand the division theorem, and as I first read about it it was the familiar experience of "what am I reading, all I see is equations and letters, and feel stressed"
So I started reading about it in other books, and it turned out it is also called the division algorithm, and it is foundational to number theory, and different authors mention different things about it, and I could find a geometric proof, with a number line, and an intuitve explanation, and another very cool analogous proof on the Eisenstein, and Gauss planes, and I can now recall those triangles and squares [Illustrated Theory of Numbers - M.H. Weissman]. And the definitions and proofs are phrased differently by everyone, nevertheless the underlying pattern is the same.
Reading mathematics books can be like solving puzzles, is another good insight for me, that understanding a paragraph, a pattern of a proof, an equation, a remark can be challenging, and itself an exercise.
writing greek letters by hand instead #
of usual letters brings variability into learning also
introducing a twist #
in the overhand knot on a stretch band creates nice patterns, and I wondered if this was a mathematical patterns. Also, I smiled that this was a good application of mathematics after all. Following the twists and curves of the knot is very pleasant for my brain.

when I notice this plant #
on my walk it reminds me of graphs:

Ellen Langer's mindfulness #
teaching is incredible, I am having so much fun.
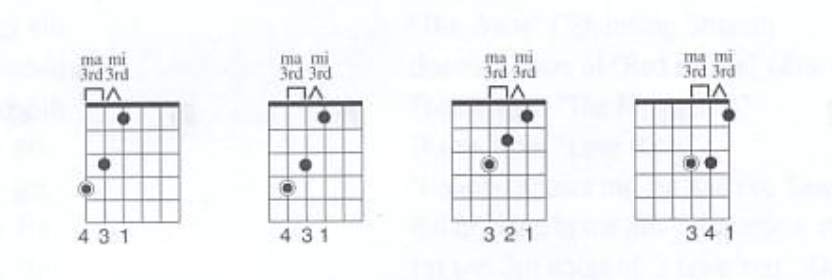
toady I learned the pattern for #
major triads starting on the thrid and fourth strings of the guitar, which I used to wonder about, as I knew the first pattern, and I went "wow". This is a bit similar to finding treasures in video games, and also I heard Paul McCartney mention in a documentary to Rick Rubin, that back in the day they had to travel to the other side of Liverpool when word got out that someone in town knew how to play a B7 chord, which they didn't. It was the missing piece from the blues pattern.
- Previous: drawing knots
- Next: some things